S. No
Model
Principle
Equation derived for turbulent mixing rate
1
Bues’ model [8] ,
The total turbulent mixing rate is formulated for two regimes. A physical model is developed for the first region i.e. bubbly-slug region and it is combined with an empirical fit for the second region i.e. annular region
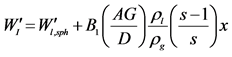
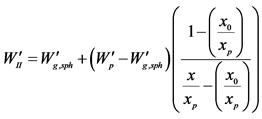
2
Kazimi and Kelly’s [9]
It is based on Bues’ model [10] which shows dependence of mixing rate on flow regimes. They proposed a correlation between the velocity fluctuation due to two phase turbulent mixing and the velocity fluctuation due to single phase turbulent mixing
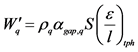 ,
,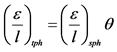
3
Kawahara et al. [10]
This model is for slug churn flow regime. In this model, the liquid phase turbulent mixing rate is the sum of three independent component mixing rate due to turbulent diffusion, convective transfer and pressure difference
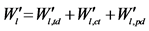 ,
,
4
Carlucci et al. [11] model
It is based on the principle that total phasic turbulent mixing rate is sum of homogenous turbulent mixing rate and incremental turbulent mixing rate
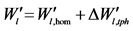 ,
,